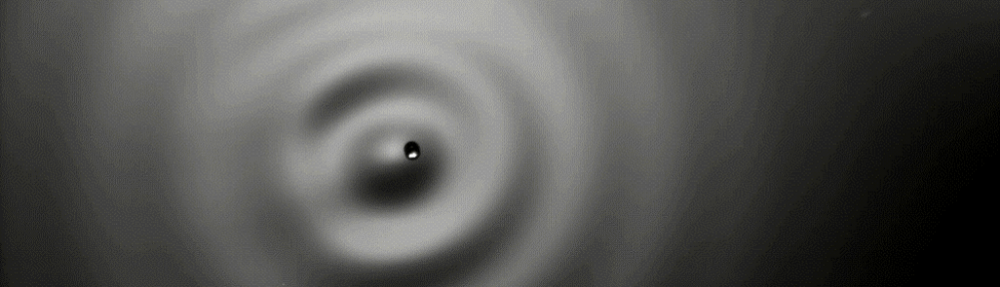
A Turing machine with wave memory

reference: « Wave-Based Turing Machine: Time Reversal and Information Erasing » by S. Perrard, E. Fort and Y. Couder, Phys. Rev. Lett. 117, 094502 (2016).

Computers possess a number discrete memories containing digital information. This quantization is fundamentally related to the fact that the information is stored using discrete entities, such as electrons for computers or DNA bases for living organisms. Is it possible to imagine a memory storing an analog information even at the elementary level? This is what we have shown with “walkers”. These are unique objects composed of a self-propelled droplet bouncing on a vertically vibrated liquid bath, driven by the waves its emits.

The droplet propagates on the bath surface piloted by the wave field created by its previous bounces. At each impact, the droplet bounces on a slanted liquid surface resulting from the presence of the wave packet. Thus, each bounce on a local slope give the droplet small horizontal kick which iteratively define its trajectory. It is important to note that the wave field itself results from the position of previous bounces of the drop and therefore from the trajectory itself. Each bounce creates a circular wave centered on the point of impact. However, unlike a pebble thrown in a pond, the emitted wave remains stationary around the impact point, sustained for a given time. This is a consequence of the vertical oscillations of the bath and the vicinity of the Faraday instability threshold. This parametric instability produces stationary waves at half the excitation frequency. Here, the propagating wave produced at each bounce by the droplet triggers locally this instability, creating a circular standing wave centered on the impact point. Since the bath excitation remains below the Faraday instability threshold, these standing waves are sustained only of a finite and tunable time which depends on the distance to the Faraday threshold. The global wave field is thus the result of the interference of these elementary circular waves deposited along the droplet trajectory. Thus the wave field holds the signature of the droplet trajectory. We have called this wave driven dynamics as a path-memory dynamics.

Walkers behavior can be analyzed in terms of information processing. They can perform the basic operations of writing, reading and erasing. These 3 basic operations are required to perform an elementary « Turing machine », the basis of all mechanical computing devices, such as a computer with memory. At each bounce, the drop writes in the wave field one elementary bit of information in the form of a circular stationary wave centered on the point of impact. This information is stored during a controllable tunable memory time. The droplet reads the information stored in the wave field as its dynamics results from the wave field.

This series of experiments conducted by Stéphane Perrard showed that the erasing operation could also be added to the walker dynamics to obtain a complete Turing machine. A small additional vertical jolt on the bath could induce a  shift between the droplet bounces and its wave field. The surface slope is changed into its opposite, and the droplet backtracks its trajectory erasing the information deposited previously in the wave field.

The fact that the elementary information is a wave, i.e. continuous, while associated to a single object, i.e. the walker, is very intriguing out of the quantum world. In classical physics, discrete objects usually are not endowed with wave characteristics. Here, the walkers possess a classical wave-particle duality which results from the intertwined dynamical interaction between the droplet and its wave field. Note that, although this duality is purely « classic », several previous studies showed that walkers displayed several features that were thaught to be part of the quantum world only.

The analysis of the walker’s dynamics in terms of information exchanges highlight the fact that this object possesses an internal wave memory. Thus, it can be considered as non-local in space and in time. The possibility for the walker to revisit its past trajectory, even when chaotic, for a characteristic time associated to its memory time raises fundamental issues about time reversal. While it is known that one can make the waves revisit their past life even in complex environments, it is much more difficult when it comes to particles because of the high sensitivity to initial conditions. Thus, a small error in the inversion of the velocity of a particle leads to rapidly diverging return paths. For walkers, their wave driven dynamics show a new behavior with the ability to be time reversed for characteristic times related to their temporal extension.

Finally, it is also interesting to look at these results in the light of the latest developments in computer science and the emergence of a new paradigm of « machine-learning » based on the use of complex networks to perform computation. These methods referred to as « reservoir computing », inspired by the brain’s ability to process information, exhibit state-of-the-art performances for processing empirical data such as speech recognition. These techniques are based on the use of complex dynamical systems with delayed feedback imitating the functioning of neuronal networks. Since space-time non-locality in processing of information is central both in these methods and in the walker’s dynamics, maybe this could open up new horizons.

This work is funded by the AXA Research Fund.