From 2D matrices to higher-order tensors for wavefront shaping
Reaching the precision limit with tensor-based wavefront shaping
Gutiérrez-Cuevas, R., D. Bouchet, J. De Rosny, and S. M. Popoff
Nature Communications 15, no. 1 (2024)
arXiv:2311.17496
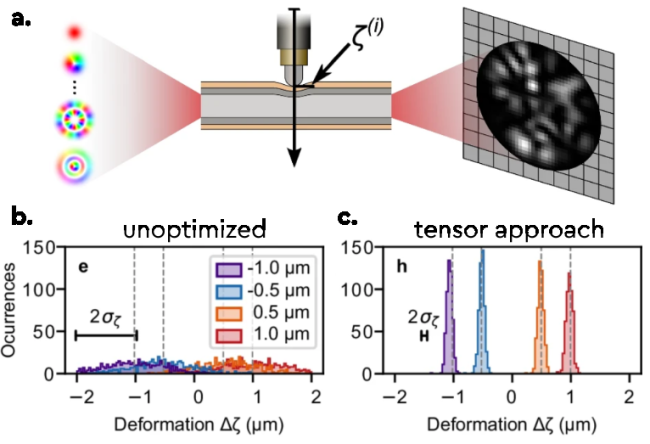
In traditional light manipulation, linear algebra solutions using matrices have been highly effective. However, these methods fall short when dealing with more than two dimensions, in particular where non-linearities come into play. This complexity requires higher-order tensors, as two-dimensional matrices can no longer describe the system. This gap has presented significant challenges in effectively managing light manipulation in complex media, such as multimode fibers or scattering media.
In this study published in Nature Communications, researchers tackled this issue by employing tensor-based wavefront shaping, extending 2D matrix techniques to higher-order tensors. This approach is crucial for systems that cannot be described by simple 2D matrices, particularly when dealing with non-linearities or when more degrees of freedom that the input wavefront need to be controlled, for instance when working with more than one wavelengths. The technique was demonstrated in a practical case involving optimal sensitivity in multimode fibers used as sensors. By only measuring output intensity, the system becomes quadratic, and optimizing both the input wavefront and output detection increases the complexity of the task. The study showed dramatic improvements in light sensitivity to minute changes in fiber configuration, enabling precise characterization of sub-micron deformations using standard fibers. This method not only enhances sensing and imaging capabilities but also holds great promise for advancements in telecommunications, showcasing the transformative potential of higher-order tensor applications in complex environments.